
The lowest y value is going to be the turning point of the variable. It looks like the y values start at 7, go down, and then start going up again. Based on the table you gave us, it looks like there is a minimum y value.
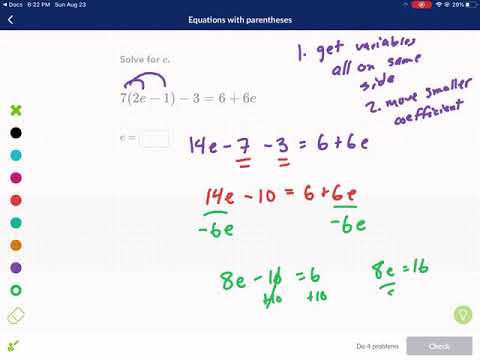
The range refers to the possible y values. Let me know if that didn't help, or if there is a specific function you are struggling with, or maybe would even like some to try out. Also, keep in mind that even if inside the absolute value bars if b was negative, outside it becomes positive. The point being you always want x by itself for this. If you ever have something like a|bx-h|+k where there is a number in front of the x you need to get rid of it if you are not aware of factoring this is what it would look like a|b||x - h/b|+k where a|b| becomes the new "a" and h/b becomes the new h, then you would solve it normally. then, since it's an absolute value function you need to know that the same line goesalong the left to make that V shape, so -5 would mean on the left down 3 and left 1. so if a was -3 that's down 3 right 1 using rise over run.
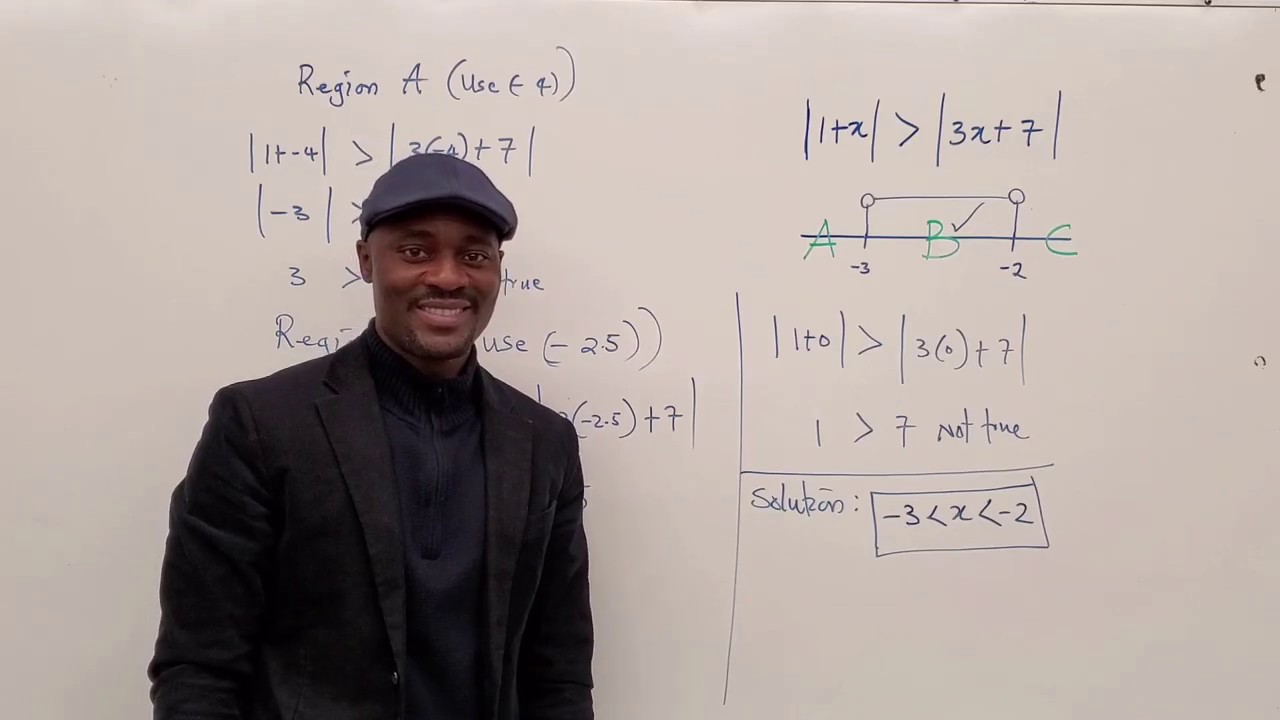
treat it like a linear equation where a is the slope. so if it helps, the x coordinate is kinda backwards.Īfter the V tip you then look at a. if you wonder why it is -5 even though we are adding 5, you just need to look at the original a|x-h|+k if we had -5 then it would be just like that, but since it is +5, we have to look at it as -5, minus negative 5.

specifically it moves the tip to (h,k) so if you have |x+5|-7 then the tip of the V shape goes to (-5,-7). Normally the tip of the V shape is at (0,0) this changes depending on h and k. a|x-h|+k Specifically you want to look at h and k first. When you have an absolute value function you want to look at what are in the places of a, h and k.


 0 kommentar(er)
0 kommentar(er)
